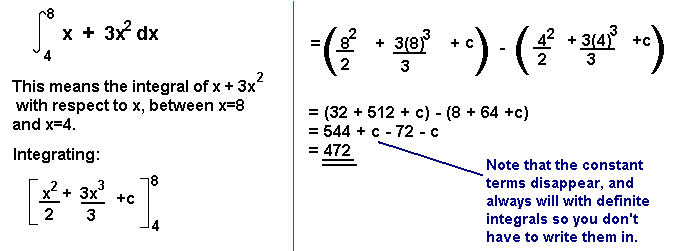
The Area Under a Curve

The area under a curve between two points can be found by doing a definite integral between the two points.

To find the area under the curve y = f(x) between x = a and x = b, integrate y = f(x) between the limits of a and b.

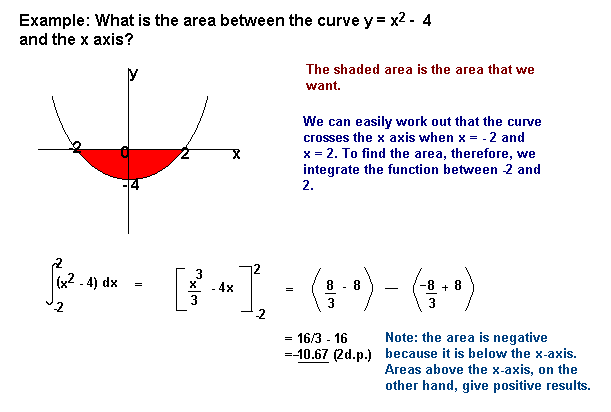
Areas under the x-axis will come out negative and areas above the x-axis will be positive. This means that you have to be careful when finding an area which is partly above and partly below the x-axis

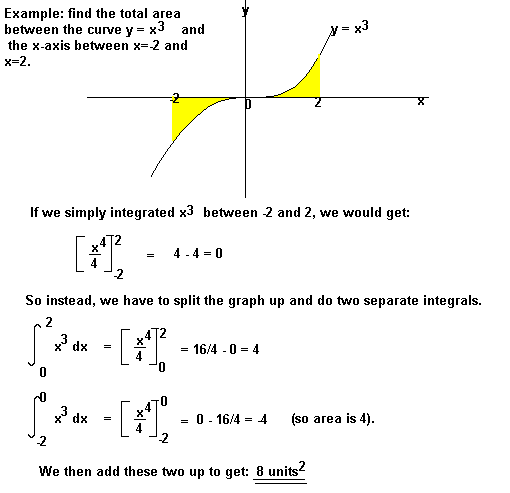
You may also be asked to find the area between the curve and the y-axis. To do this, integrate with respect to y.

Find the area bounded by the lines y = 0, y = 1 and y = x²